The Beauty of Fractals

Fractals are fascinating mathematical objects that possess a captivating beauty. They’re not just pretty pictures; they’re a glimpse into a world of infinite detail and self-similarity, a world where patterns repeat themselves endlessly at different scales. It’s like zooming into a picture, only to find the same intricate details repeated again and again, forever.
The Origins of Fractal Geometry
The study of fractals, known as fractal geometry, emerged in the 20th century, with the pioneering work of Benoit Mandelbrot, a Polish-born mathematician. He coined the term “fractal” in 1975, drawing inspiration from the Latin word “fractus,” meaning “broken” or “fragmented.” Mandelbrot’s work challenged the traditional Euclidean geometry, which focuses on smooth, continuous shapes. He recognized the importance of irregular, fragmented shapes found in nature, and his research opened up a whole new realm of mathematical exploration.
Fractals in Nature
Fractals aren’t just abstract mathematical concepts; they’re found all around us in the natural world. Look closely at a coastline, and you’ll see the same jagged, irregular patterns repeating at different scales. Snowflakes, with their intricate six-pointed designs, are another example of nature’s fractal beauty. Even the branching patterns of trees and the veins in our leaves exhibit fractal properties. These natural examples demonstrate that fractals are not just mathematical curiosities; they’re fundamental building blocks of the world we live in.
Types of Fractals
Fractals come in a wide variety of shapes and forms, each with its own unique properties. Here’s a glimpse into some of the most famous fractal families:
| Fractal | Properties | Visual Representation |
|---|---|---|
| Mandelbrot Set | Defined by a simple equation, the Mandelbrot set is a stunningly complex fractal that features infinite detail and intricate boundaries. | [Describe the Mandelbrot set, its intricate shape, and color variations. Mention the black region, the “heart” of the set, surrounded by colorful, complex structures. Highlight the infinite zoom capability, revealing more detail at each magnification.] |
| Julia Sets | Similar to the Mandelbrot set, Julia sets are defined by a related equation, but they exhibit a wider range of shapes and patterns, depending on the chosen parameter. | [Describe Julia sets, highlighting their variety in shape and color. Mention the “connected” and “disconnected” Julia sets, with the latter often resembling a dust cloud. Emphasize the sensitivity to initial conditions, leading to drastically different outcomes for slightly different starting values.] |
| Sierpinski Triangle | This fractal is constructed by repeatedly removing equilateral triangles from an initial triangle, creating a pattern of smaller and smaller triangles. | [Describe the Sierpinski triangle, highlighting its self-similarity and the endless repetition of the triangular pattern at different scales. Mention its “empty” spaces, which are as important as the filled triangles in defining its structure.] |
| Koch Snowflake | This fractal is formed by repeatedly adding equilateral triangles to the sides of an initial triangle, creating a snowflake-like pattern with infinite perimeter and finite area. | [Describe the Koch snowflake, highlighting its sharp, pointed edges and the way it grows infinitely complex as more triangles are added. Mention its fascinating property of having an infinite perimeter, even though it encloses a finite area.] |
Unveiling the Mandelbrot Set: Fractals And Chaos The Mandelbrot Set And Beyond
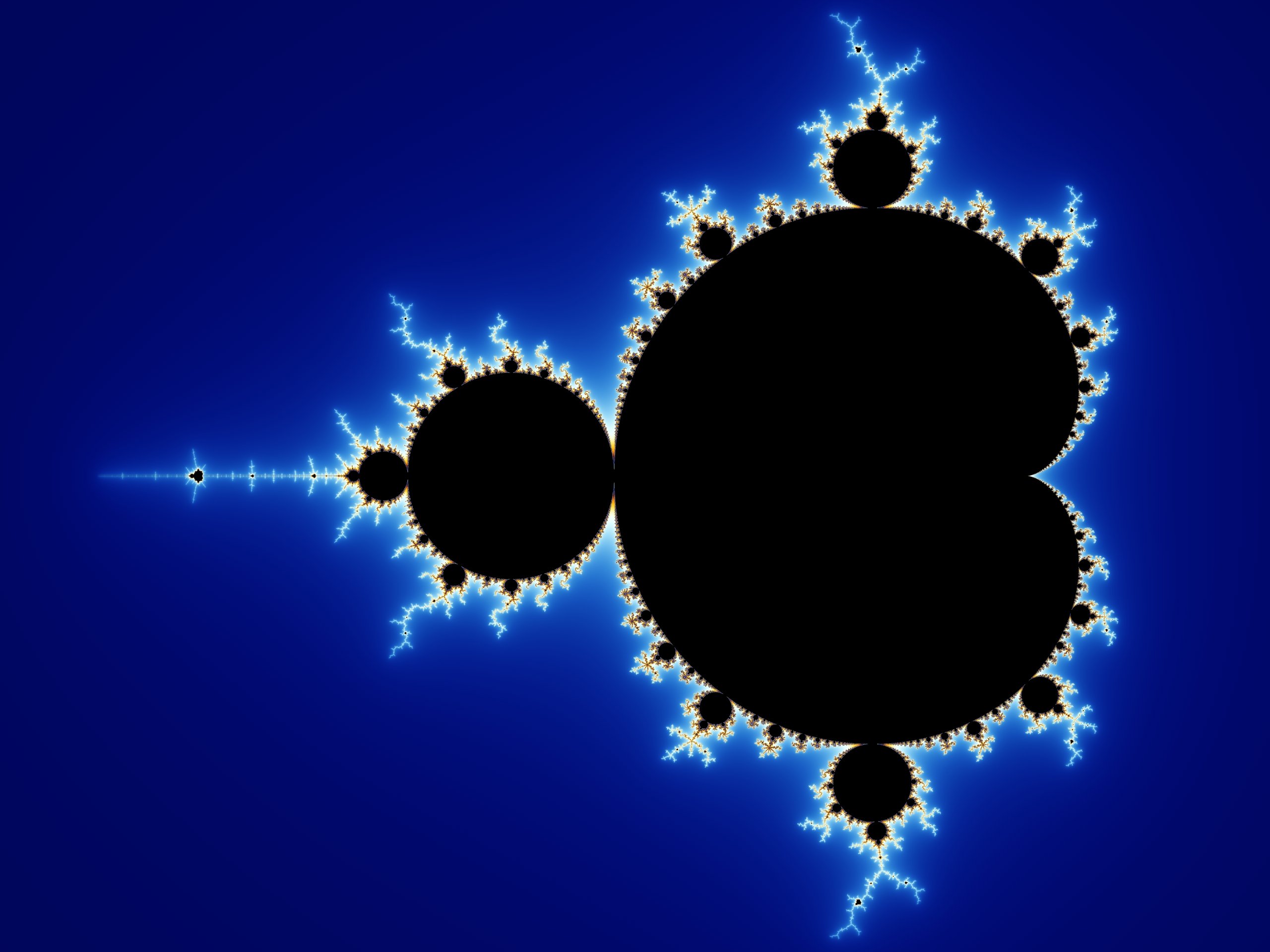
Prepare to have your mind blown, because the Mandelbrot set is a wild ride into the world of fractals. It’s a captivating example of how complex and beautiful math can be, and it’s got more twists and turns than a rollercoaster at a carnival.
The Mandelbrot set is a fascinating fractal generated by a simple yet powerful mathematical formula. It’s all about iteration, which is like playing a game of mathematical tag. You start with a number, plug it into the formula, get a new number, and then plug *that* number back in. You keep repeating this process, and the Mandelbrot set is born from the results.
The Formula and Iterative Process
The magic formula behind the Mandelbrot set is:
zn+1 = zn2 + c
Where:
* z is a complex number that starts at 0.
* c is a complex number that represents a point on the complex plane.
To generate the Mandelbrot set, we iterate this formula for each point (c) on the complex plane. We check if the absolute value of z stays bounded (meaning it doesn’t get too big) as we iterate. If it does stay bounded, then the point c belongs to the Mandelbrot set.
Escape Time and Color Patterns
Now, here’s where things get really interesting. The “escape time” is how many iterations it takes for the absolute value of z to exceed a certain threshold (usually 2). This escape time is what gives the Mandelbrot set its mesmerizing colors and patterns. If a point escapes quickly, it’s assigned a lighter color. If it takes a long time to escape, it gets a darker color. And if it never escapes, it’s part of the black core of the Mandelbrot set.
Interesting Features, Fractals and chaos the mandelbrot set and beyond
The Mandelbrot set is packed with fascinating features that make it a playground for mathematicians and artists alike. Here are a few highlights:
- The “Sea Horse Valley”: This is a beautiful, elongated region within the Mandelbrot set that resembles a seahorse. It’s a perfect example of how the Mandelbrot set exhibits self-similarity, meaning that smaller parts of the set look like larger parts.
- “Period Doubling”: As you zoom into the Mandelbrot set, you’ll notice a pattern of repeated structures. The “period doubling” phenomenon refers to how these structures double in number as you zoom in further. It’s like a mathematical echo chamber.
Chaos and its Connection to Fractals

Imagine a butterfly flapping its wings in Brazil, causing a hurricane in Texas. Sounds crazy, right? But that’s the essence of chaos theory – seemingly insignificant events can have massive, unpredictable consequences. And believe it or not, fractals are deeply intertwined with this wild world of chaos.
The Nature of Chaos
Chaos theory describes systems that are incredibly sensitive to initial conditions. Even the tiniest change can lead to drastically different outcomes. Think of it like trying to predict the weather – a slight shift in air pressure can completely alter the forecast.
Chaotic Systems in Nature
- Weather Patterns: The atmosphere is a chaotic system, making long-term weather predictions incredibly difficult. The complex interactions of air pressure, temperature, and humidity create patterns that resemble fractals, with swirls and eddies at different scales.
- Population Dynamics: The growth and decline of populations can be chaotic, influenced by factors like food availability, disease, and predator-prey relationships. Fractal patterns can emerge in the distribution of species over time and space.
- Fluid Flow: The movement of fluids like water and air can exhibit chaotic behavior. Think of turbulent rivers or the swirling patterns of smoke. Fractals play a role in the formation of these intricate structures.
The Lyapunov Exponent
The Lyapunov exponent is a mathematical tool used to quantify the degree of chaos in a system. It measures how quickly nearby trajectories diverge from each other. A positive Lyapunov exponent indicates chaos, while a negative one suggests stability.
“A butterfly flapping its wings in Brazil can cause a hurricane in Texas.” – Edward Lorenz
Visualizing the Connection
Imagine a beautiful, intricate fractal like the Mandelbrot set. Now, picture a drop of ink falling onto its surface. The ink will spread out, following the complex pathways of the fractal, creating a chaotic pattern that mirrors the unpredictable nature of the system. This visualization illustrates how chaos and fractals are interconnected, each influencing the other.
Fractals and chaos the mandelbrot set and beyond – The Mandelbrot set, a stunning example of fractal geometry, reveals infinite complexity within a finite space. Its intricate patterns echo the natural world, from the branching of trees to the swirling patterns of clouds. This same sense of intricate design can be found in the craftsmanship of a wood designs square table and chair set , where the grain of the wood itself reveals hidden patterns and textures.
Just as the Mandelbrot set expands endlessly into the depths of the complex plane, so too does the beauty of natural forms inspire endless creativity in human design.
Just as the Mandelbrot set reveals infinite complexity within a seemingly simple equation, the right restaurant table chair set can transform a dining space into a haven of comfort and style. Every detail, from the grain of the wood to the curve of the chair back, adds to the overall aesthetic, creating a harmonious environment where even the most mundane meal becomes a moment of enjoyment.
Similarly, exploring the intricate patterns of fractals and chaos invites us to see the beauty in the unexpected, the order in the seemingly random, and the infinite potential within the seemingly finite.
